Timing Structure and Inhomogeneities of the MAGIC Camera

It was checked whether other signal extractors reduce/do not show/enhance the timing-camera nonuniformity correlation.  The other signal extractors used were the  "Sliding Window" with a 6(8) slice integration window for the high (low) gain and the Spline integrating over 1 (1.5) slices in the high (low) gain. The extraction window edges of the pulse position check option were set to the same values as for the Digital Filter (-2.5/+4.5+integration size from the mean) .  The result can be summarized as follows:

• Although other signal extractors show relatively large differences in the arrival time and signal reconstruction, the overall features do not change, i.e, also there a correlation of the camera nonuniformity to the window position and size can be observed.
  

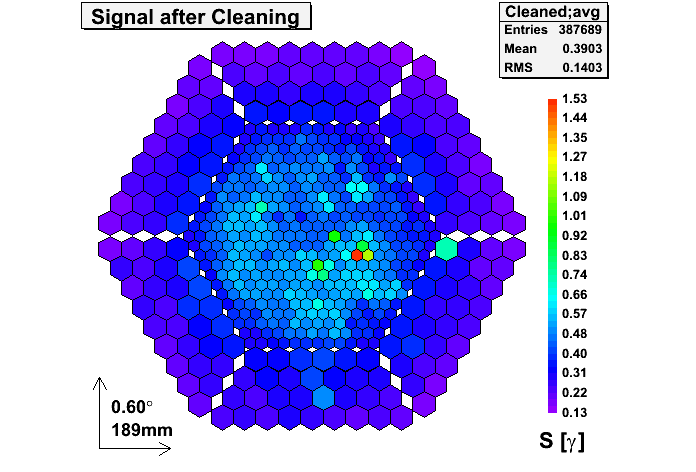
• As an example,  the camera plots of the event and charge distribution are shown here for the night of 2005/06/01 using the spline extractor. Compared with the Digital Filter, the inhomogeneities seem to be a little less pronounced.
  
• The same example was used for a comparison of Sliding Window and Digital Filter. With  the position check on, the Sliding Window gives a much smoother picture than the Digital Filter. The improvement by switching the pulse position check off is much smaller, though.
• For the night of 2005/05/07, where the arrival times are very early, the Sliding Window produces a slightly smoother camera distribution than the two other extractors. Here you can find a comparison of the Sliding Window with the Spline extractor , without the pulse position check applied.
  

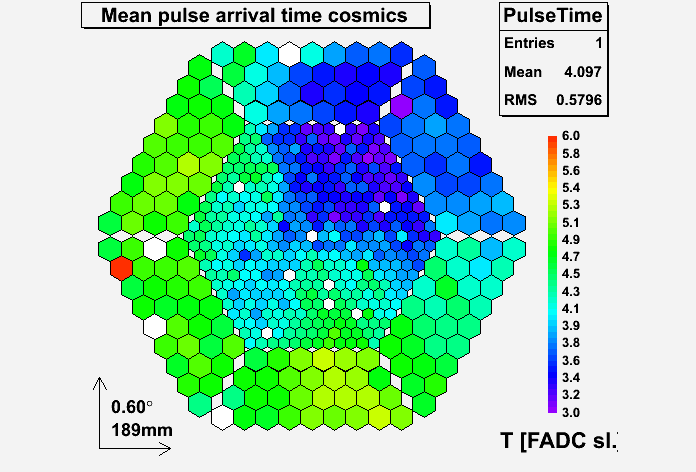
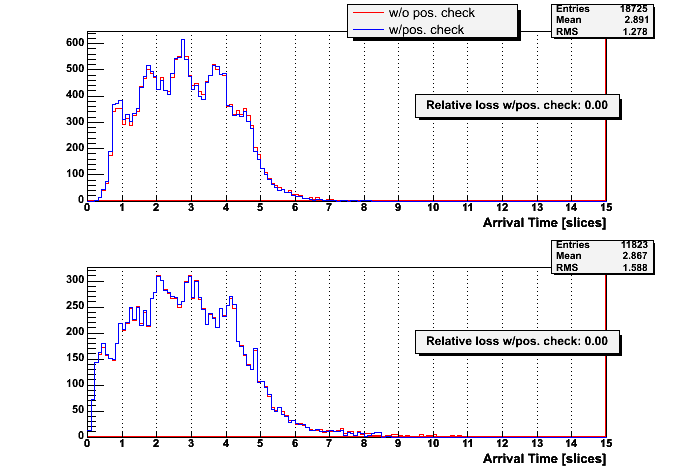
• Another issue is the determination of the arrival time itself. Below, the average arrival time distributions are shown for 2005/06/01 using the Spline and the Sliding Window extractors.
• 
  
• The comparison of these plots with each other and with the corresponding plot for the Digital Filter shows that the finger like structure of the arrival time distribution might be an effect of the extractor. The fingers of the Spline are shifted with respect to the Digital Filter, and "smeared out" or not there for the Sliding Window.
• The finger structure on the other hand seems to be correlated with the extracted charge distribution. This can be seen from the plot showing the average reconstructed charge  in N(pe) per inner pixel as a function of the reconstructed arrival time for the Digital Filter (red), the Spline (blue) and the Sliding Window (green). For the Spline and the Digital Filter the "peaks" in this distribution are clearly anticorrelated to the fingers in the time distribution.
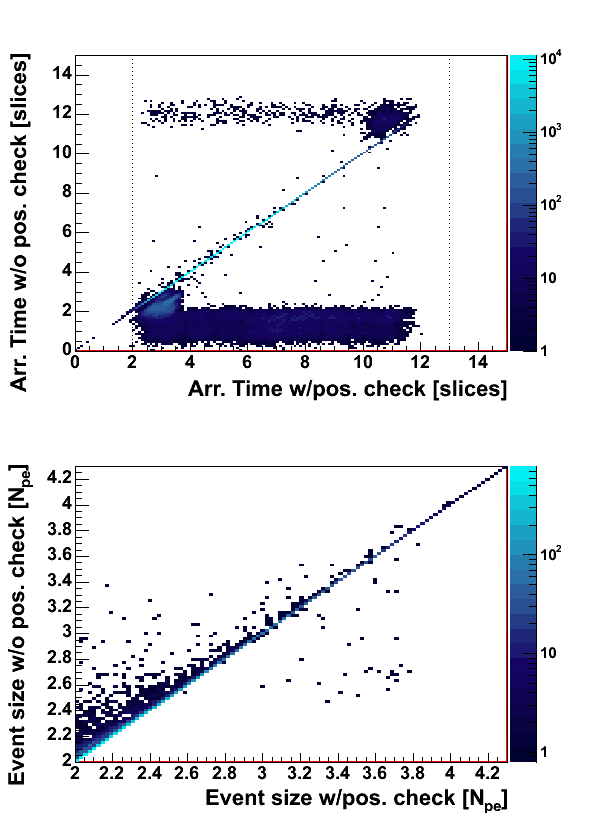
• The reason for this behavior can be seen from the scatter plot of the N(pe) per inner pixel vs. the arrival time, shown here for all three extractors. The two upper plots show the distributions for Digital Filter and Spline, respectively. At the  edge of a time slice (i.e., the "dips"), there are not only less events than in the middle (at the "bumps"), but also clearly low charge events missing. This correlates the "dips" in the  scatter plot with the "bumps" in the profile plot, i.e., with the higher average reconstructed charge. On the other hand we see in the arrival time distribution, that the "peaks" are located at the edges of a slice. This merely shows that events with small charges are more likely reconstructed in the middle of a slice than on the edge.
•  For the sliding window there is no obvios correlation to the time distribution.
  
• There is an overall correlation of the average reconstructed charge of all extractors with the arrival time, giving smaller sizes for the early and late times. This may be partially due to the inefficiencies of the extractors to reconstruct early/late pulses, but may as well be partially generated by physics (shower development).

-> Back to top
-> Back to Other Signal Extractors

Camera plots for 2005/06/01 using Spline (left: with pulse position check, right: without pulse position check)

Camera plots for 2005/06/01 using Sliding Window (left: with pulse position check, right: without pulse position check)

Camera plots for 2005/05/07 using Sliding Window (left) and Spline (right) without pulse position check

The average arrival time per event on 2005/06/01 using Spline  (top: inner, bottom outer camera)

The average arrival time per event on 2005/06/01 using Sliding Window  (top: inner, bottom outer camera)

The Average Reconstructed Charge per Pixel as a Function of the Arrival Time for Three Extractors

The Reconstructed Charge per Pixel as a Function of the Arrival Time for Three Extractors

-> Back to top

---

-> Back to top

Size Dependence of Inhomeneities

The influence of a lower event size cut has been investigated. (Note that the event size used here is not the same as the one used in the hillas files, as there is no image cleaning applied). Below the camera plots are shown for 2005/06/01, using a size cut of

1. 250 N(pe)
2. 500 N(pe)
3. 1000 N(pe)
4. 4000 N(pe)

The camera images seem to be a little bit smoother for size cuts above 500, however, the nonunoformity rermains to some degree. Between size cuts of 500 and 2000 the picture does not change much, at size > 4000 the nonuniformity gets stronger, though.
The logarithm of the average event size is shown below against the average arrival time of the event. Also there, a strong dependence of the average event size on the arrival time can be seen, especially at the early arrival times. The rise at late times might as well be due to physics reasons. This needs to be checked further.
 
-> Back to top
-> Back to Size Dependence

Camera plots for 2005/06/01 with size>250 (left) and size>500 (right) pe without pulse position check

Camera plots for 2005/06/01 with size>1000 (left) and size>4000 (right) pe without pulse position check

Average of event size (above 100) vs. the average arrival time of the event (night of 2005/06/01)

-> Back to top

---

-> Back to top

Conclusions

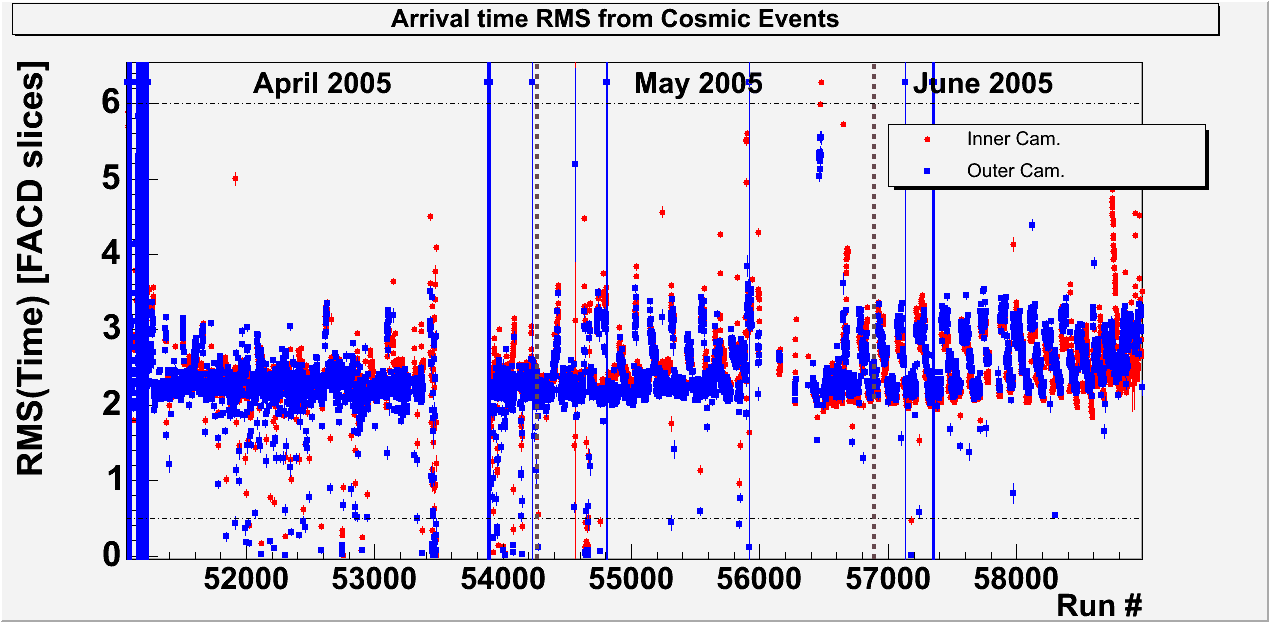
The inhomogeneity of the MAGIC camera with respect to the event and charge distributions seems to be generated by the spatial distribution of the arrival times in the camera. This in turn is caused by the setup of the  trigger delays for the different macro cells. The arrival time  distribution generates arrival time differences between events of up to 2 slices, depending on the position in the camera where the trigger was generated.  Therefore, even with moderate mean arrival times (>4 FADC slices), the arrival time RMS is relatively large. This means that always some part of the events has very early (resp. late) arrival times and is not being reconstructed correctly, i.e, the events are lost or  reconstructed with a too small charge.
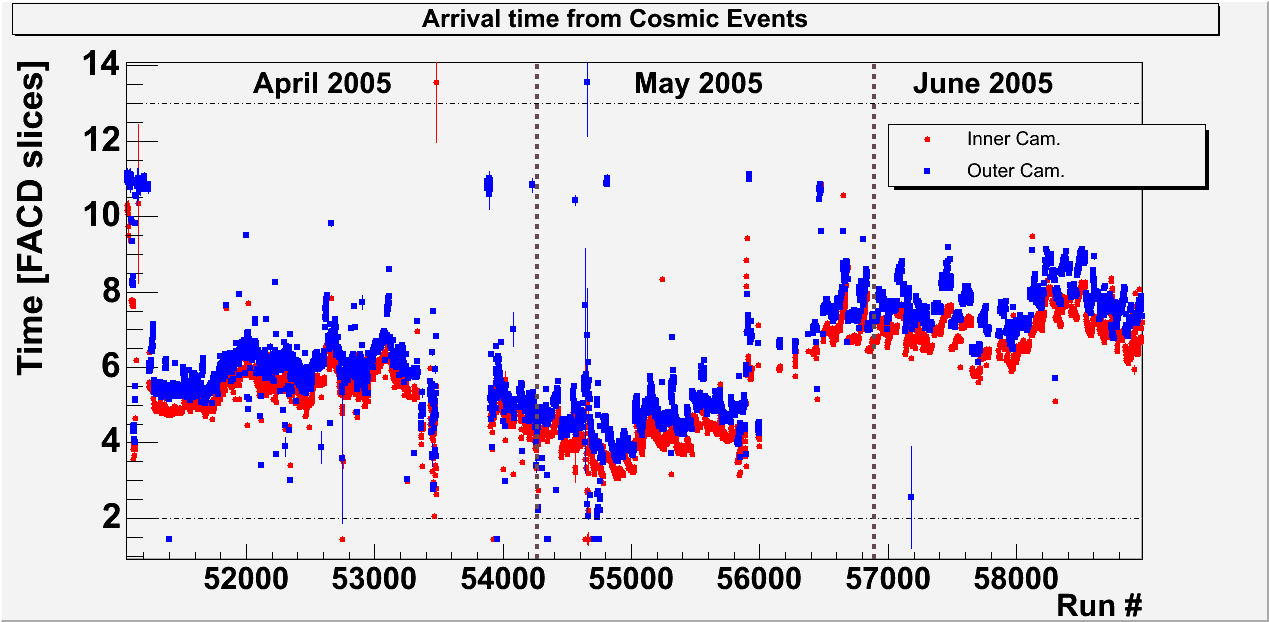
This effect is even stronger, if the mean arrival times are in general very early (late), as it happened for instance very often in May.
The effect is usually enhanced by the software (callisto) if the default pulse position check is switched on. It should be clear that shifting the lower edge of the signal extraction window 2 slices up (typical for the pulse position check) is basically the same as shifting the mean of the arrival time 2 slices down.

What should be on the "To Do List" is the following:

• Fix the trigger delays to even out the arrival time differences in the camera
• For the time being:
• Switch off the pulse position check when calibrating data
• Careful with data from Periods with early/late mean arrival times and/or large arrival time RMS. The effects should be evaluated using Monte Carlo.
• Other featurre seen during this study should be clarified as well:
• The "fingers" in the arrival time distribution of pixels/events for the Digital Filter and Spline extractors
• The dependence of the  reconstructed pixel charge on the (reconstructed) position within a slice,  which seems to be correlated to the "fingers"
  

-> Back to top

patty@mppmu.mpg.de